Print this page

Topic: Properties of Angles and Shapes (Higher - Unit 3)

Specification References: G1.2 G1.2 Understand and use the angle properties of parallel and intersecting lines, triangles and quadrilaterals.

Candidates should be able to:

• understand and use the angle properties of parallel lines
• recall and use the terms, alternate angles, and corresponding angles 
• work out missing angles using properties of alternate angles and corresponding angles
• understand the consequent properties of parallelograms
• understand the proof that the angle sum of a triangle is 180^o
• understand the proof that the exterior angle of a triangle is equal to the sum of the interior angles at the other two vertices
• use angle properties of equilateral, isosceles and right-angled triangles
• use the angle sum of a quadrilateral is 360^o

Notes

Candidates should be aware that colloquial terms such as ‘F angles’ or ‘Z angles’ should not be used.

Candidates should know the names and properties of isosceles, equilateral, right-angled and scalene triangles.  

Examples

1. 
   
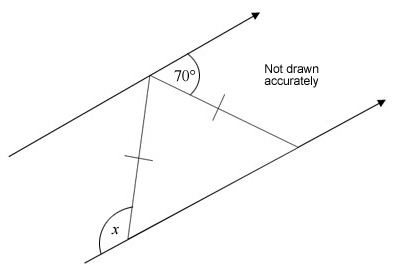
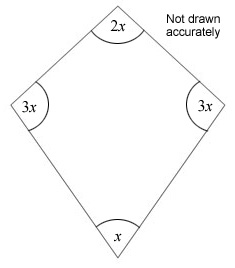
   Work out the size of x.
   You must explain any properties that you have used to obtain your answer.
   

view more examples